Home  ›  alternate interior angles theorem  ›  alternate interior angles theorem parallelogram  ›  alternate interior angles theorem triangles  ›  consecutive exterior angles theorem  ›  consecutive interior angles theorem converse  ›  consecutive interior angles theorem definition  ›  consecutive interior angles theorem examples  ›  consecutive interior angles theorem proof

Consecutive Interior Angles Theorem

Written By Sunday, July 31, 2022 Add Comment Edit

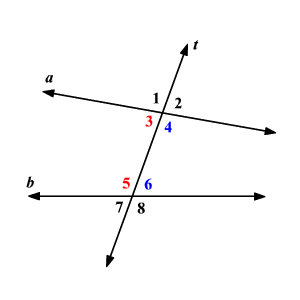
Consecutive Interior Angles Theorem. To help you remember: the angle pairs are Consecutive (they follow each other), and they are on the Interior of the two crossed lines. We will show that if the consecutive interior angles on the same side of a transversal.

mrwadeturner / T2 Alt Exterior and Consecutive
mrwadeturner / T2 Alt Exterior and Consecutive (Gertrude Patrick)
When two lines are crossed by another line (called the Transversal ): The pairs of angles on one side of the transversal but inside the two lines are called Consecutive Interior Angles. The Consecutive Interior Angles Theorem states that if two parallel lines are cut by a transversal, then each pair of alternate interior angles is congruent. From the properties of the parallel line, we.

The Consecutive Interior Angles Theorem states that if two parallel lines are cut by a transversal, then each pair of alternate interior angles is congruent.

The consecutive interior angles theorem states that if a transversal passes through two parallel lines, it makes two pairs of consecutive interior angles that are supplementary.

Consecutive Interior Angles: Definition & Theorem - Video & Lesson ...

Consecutive Interior Angles Examples - img-napkin

Converse Of Same Side Interior Angles Theorem Proof | Review Home Decor

Consecutive Interior Angles Theorem

Consecutive Interior Angles Converse Theorem

Corresponding Angles Converse Example - diocartoon

mrwadeturner / M1 Consecutive and Alternate Exterior Angles

Alternate Interior Angles Congruent | Cabinets Matttroy

PPT - Section 5.1 - Parallelograms PowerPoint Presentation - ID:4297759

Theorem 3 4 Alternate Interior Angles Converse | Review Home Decor

Same Side Interior Angles Theorem Converse | Review Home Decor

Ilectureonline

Malcolm has a Master's Degree in education and holds four teaching certificates. Here's how you prove the Consecutive Interior Angles Theorem: The consecutive interior angle theorem determines the relationship between the successive interior angles in a given sequence. Do you know the difference between interior and exterior angles?

Judul: Consecutive Interior Angles Theorem
Rating: 100% based on 788 ratings. 5 user reviews.
Cheryl Mckenzie

Thank you for reading this blog. If you have any query or suggestion please free leave a comment below.

0 Response to "Consecutive Interior Angles Theorem"

Post a Comment

Subscribe to: Post Comments (Atom)